Navier stock equation

It’s the fundamental equations describing fluids dynamics, the Navies Stocks equations are famously challenging to understand, mainly is essentially a form of newton’s second law: the acceleration of the body times its mass equals to the sum of the forces acting on it, and mass conversation. For compressible and Newtonian fluid, these equations are presented as below:

\begin{align} \nabla (V) &= 0 \newline \frac{D (\rho V)}{Dt} & = -\nabla P+\mu \nabla V^2 + \rho g \newline \frac{\partial \rho V}{\partial t}+ \nabla (\rho V^2) & = -\nabla P+\mu \nabla V^2 + \rho g \end{align}

Physically, it’s easy to understand the meaning of each term:

• $\frac{\partial \rho V}{\partial t}+ \nabla (\rho V^2) $ is analogues to mass times acceleration

• $\nabla P $ is the pressure forces

• $\mu \nabla V^2 $ is the viscous forces

• $\rho g $ is external forces ad gravity for instance.

These equations can be used for almost any problem involving fluid: as aerodynamics of formula 1, designing supersonic aircraft or Fixed-wing aircraft, studding the motion of the blood inside the body, pollution modeling, climate modeling and cloud motion, ocean and river flows… all these problems satisfy these equations.

However, mathematically, we don’t understand these equations, in the sense that, as mathematician, when you want to solve a set of differential equation like $\dot Y=a*Y+b$, you want that these equations satisfy some particular properties:

• The solution must exist.
• The solution must be unique according to the boundary conditions.
• The solution must be robust (if you made a little or tiny change in the boundary conditions the result must change smoothly)

Many mathematicians and smart peoples since the previous century worked to achieve this step, unfortunately they can’t prove the existence of a robust solution all the time, given the velocity or pressure as boundary conditions. That’s way in 2000 these equations was listed on the Millennium Prize Problems, and the genius who solves this problem will be rewarded by 1 Millions €.

Hence, in order to deal to this problem, the CFD codes nowadays, that have been developed since 1960 to solve these equations numerically to simulate a fluid motion, try to do some simplifications or make some assumptions like using the Reynolds-averaged method and feeding up these equations by complementary models ( large Eddy simulation,Standard K-ε, Detached Eddy Simulation…) , in order to presents the turbulence phenomena that can occur, based on the studied problem, the circumstance of the flow, and the boundary condition. In fact, turbulence remains one of the greatest unsolved problems in physics, and one the key to understand this unsolvable math problem is to understand the turbulence, which is the chaotic, eddy and the random motion of the fluid particle, this fluid motion is so difficult to understand and model. Furthermore, it depends strongly with the fluid velocity and the fluid viscosity.

That’s way, scientists introduce the Reynold number which can be interpreted as the ratio between the inertia forces (created by the fluid velocity) and the by fluid friction forces (related to the fluid viscosity) when the Re number in less that 4000 the fluid motion is characterized with the laminar motion, the fluid moves smoothly and it’s easy and reasonably the predict and understand the fluid motion, however, when the Re number is higher the turbulence motion appears and the physic phenomena is difficult to understand.

An intuitive experience that highlights the turbulence motion due to the boundary layer separation is presented in this video (2.22 to 4.22)

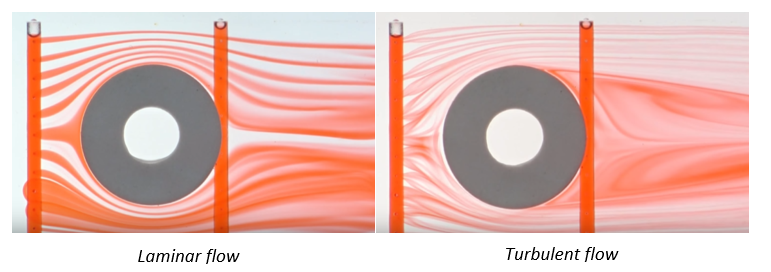
Most scientist think that solving these mysterious equations will rely on predicting the statistical evolution of turbulent flows, and increase computing power. Extremely high-speed computer simulations of turbulent flow could help to identify patterns that could lead to theory that organize and unifies predictions across different situations. By reaching this breakthrough, the understanding of turbulence could have huge positive impacts, as better prepare and predict weather disasters, the flow of blood through the cardiovascular system, the airflow over an aircraft wing…..